Properties of the 2D Fourier Transform

The Fourier Transform of the different 2D images were taken and the results are shown in the figures below.

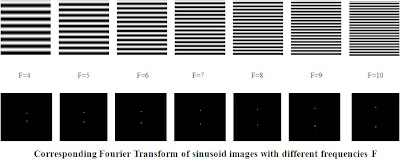
The next figures show the images of the sinusoid with various frequencies. It is highly seen that as the frequency of the sinusoid is increased, the distance between the two points corresponding to the frequency in the Fourier domain also increases. The points represent the transformation of the continuous signal to discrete signals upon undergoing Fourier Transform.

When a constant bias is added to the sinusoid, another peak located at the center between the two points. This is similar to a dirac-delta function centered at the middle of the two mirror imaged frequencies. In order to determine the peak frequencies, the 3D plot is performed.

The result of the Fourier Transform of the additional non-constant bias to the sinusoid is the appearance of other peaks that are located at the frequency values of the bias. Note that for every frequency, there are two peaks found because the Fourier transform consists of complex numbers, so the absolute value is taken. Thus, the negative and positive values are considered.

When the sinusoid is rotated, the corresponding Fourier transformed image is also rotated, the angle of which depends on the rotation angle of the input. This is observed from the figures below.

The next two figures are the image of the Fourier transform (right) of the product of two sinusoid running in X and Y axes.

The last four figures correspond to the addition of a non-constant bias at different rotation angles to the product of the two sinusoids above. The bias used in the first two images is a sinusoid with frequency F=20 and rotated to theta=60 degrees. The last two shows the image of the input sinusoid biased with another sinusoid at F=18 and theta=90degrees. It was predicted that another two points will appear on the FT and these are rotated in angles equal to the rotation angle used.

In this activity, I am giving myself a grade of 10 since all the tasks are completed and with desirable and correct results.

I would like to thank Gary Garcia and Winsome Rara for helping me improve my images.